Chapter: Discrete time dynamics
(ChaosBook.org blog, chapter Discrete time dynamics) — Predrag Cvitanovic 2009-02-11 12:55
Section: Poincaré sections
 If GaTech world domination is to be maintained, we need to start taking Poincaré sections of unstable manifolds. Make sure you understand chapters on Discrete time dynamics, Local stability and Cycle stability. — Predrag Cvitanovic 2009-02-11 12:55
If GaTech world domination is to be maintained, we need to start taking Poincaré sections of unstable manifolds. Make sure you understand chapters on Discrete time dynamics, Local stability and Cycle stability. — Predrag Cvitanovic 2009-02-11 12:55
 Constructing Poincaré sections and return (or forward) maps is not such a big deal - it's just that no masters of plumbing listen to my pleas. Jul 6 2006 I got Halcrow to give it a try:
Constructing Poincaré sections and return (or forward) maps is not such a big deal - it's just that no masters of plumbing listen to my pleas. Jul 6 2006 I got Halcrow to give it a try:
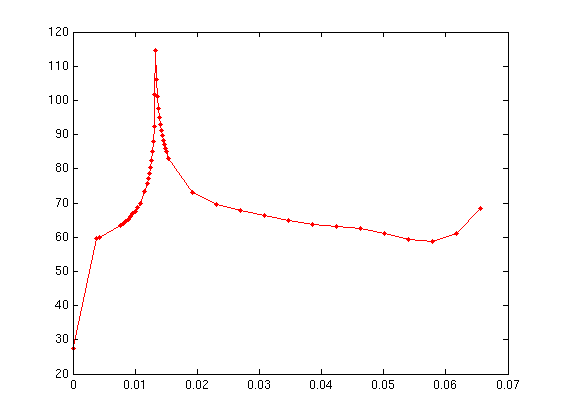 Poincaré return time to first intersection with a Poincaré section, normal to one of the eigenvectors corresponding to the most unstable complex pair of eigenvalues of the upper branch.
~~CL~~
Poincaré return time to first intersection with a Poincaré section, normal to one of the eigenvectors corresponding to the most unstable complex pair of eigenvalues of the upper branch.
~~CL~~
It was right in spirit. It was wrong in detail: Jonathan simply used a finite length, straight line segment of the linear eigenvector instead of the curved unstable manifold for initial points, and the axes scales are - as is the custom among the nonlinear graduate students - profoundly mysterious. A return map maps a curvilinear segment labeled by arclength  into
into  , i.e., the graph should be a square, with the same units on both axes. “Poincaré return time” is something else.
, i.e., the graph should be a square, with the same units on both axes. “Poincaré return time” is something else.
He never listened to me again, so there it stands. John G's periodic orbit P47.18 in the W03 cell presumably sits very nicely on it.
With Y. Lan I had a bit more luck. He resisted for 6 years or so, but than his wife told him that thesis should be finished this semester and he relented. His Kuramoto-Sivashinsky (“fluid dynamics” in one dimension) plots all like the usual nonlinear garbage:
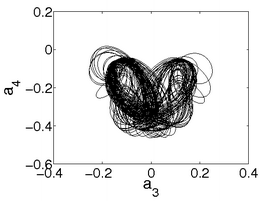 projected on random coordinates (first Fourier modes) until he relented and plotted the unstable manifold of the “upper branch,”
projected on random coordinates (first Fourier modes) until he relented and plotted the unstable manifold of the “upper branch,”
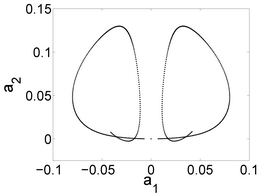 and relented even further and plotted the
and relented even further and plotted the  discrete symmetry quotiented return map:
discrete symmetry quotiented return map:
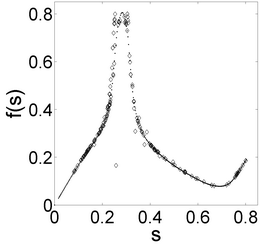 And - (the mystery hidden from human eye by being written in the ChaosBook!) - very many periodic orbits followed, labeled by a ternary alphabet.
And - (the mystery hidden from human eye by being written in the ChaosBook!) - very many periodic orbits followed, labeled by a ternary alphabet.
So I know you can do it, if you get your mind to it.
~~DISCUSSION~~
