gibson:teaching:spring-2015:math527:finaloutline
Math 527 Outline of topics for final exam
Think of the final exams as 2/3 (exam1 + exam2 + exam3 + systems of equations). That's about right to fit in the allotted two hours. There will be around eight problems chosen from this outline of topics.
- 1st order equations
- separable
- exact
- 1st order linear
- 2nd and higher-order equations
- homogeneous constant coefficient (
 is your friend)
is your friend) - judicious guessing
- variation of parameters
- power series
- Laplace transforms
- definitions, properties, and transforms & inverses of simple functions
- s-translation, t-translation
- Heaviside and Dirac delta functions
- convolution
- Systems of equations
- matrices, vectors,
 problems, and determinants
problems, and determinants - the eigenvalue problem, how it arises from the ODE
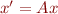
- how to solve systems of ODEs with
- real eigenvalues, distinct
- real eigenvalues, repeated
- complex eigenvalues (solutions expressed in both complex and real-valued forms)
- phase portraits
gibson/teaching/spring-2015/math527/finaloutline.txt · Last modified: 2015/05/07 09:38 by gibson
